Catenary
Object type: Plane curve
Definition
The catenary is the graph of the hyperbolic cosine, or, more generally, the function $x \mapsto a \cosh {\left(\frac{x}{a}\right)}$ for some $a > 0$. In the image below, $a = 5$.
Curvature
The curvature function for the catenary (parameterised using $x$ as parameter) is the bump function $$\kappa(x) = \frac{1}{a} \text{sech}^2 {\left(\frac{x}{a}\right)}. $$
Examples
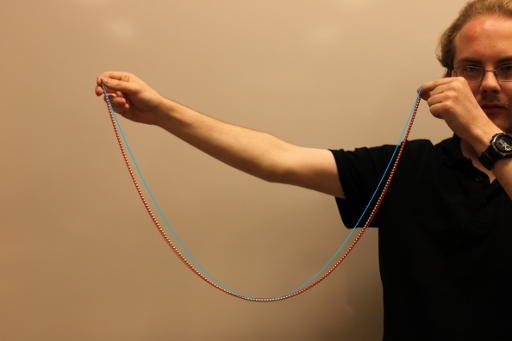
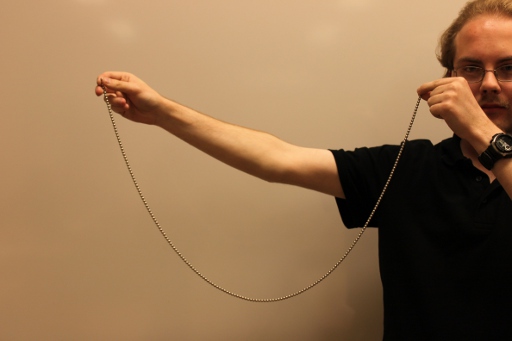
An ideal chain (cable, rope, wire) placed in a (constant) gravitational field suspended at its ends will obtain the shape of the catenary. Below this is demonstrated by the author himself.
Let us superimpose one parabola (blue) and one catenary (red):